Guest blogger Juan Camilo Acevedo is part of the University of Chicago’s Center for Elementary Mathematics and Science Education (CEMSE) digital team, where he develops Sketchpad-based activities for Everyday Mathematics. Currently, he teaches undergraduate language classes at the University of Chicago and is writing his doctoral dissertation on Digital Humanities. Juan holds a BA in mathematics from the University of Los Andes (Bogotá). He enjoys cross-disciplinary approaches to teaching and learning as well as the use of technology to enhance pedagogical experiences.
A great feature of Dynamic Geometry software like The Geometer’s Sketchpad is that it allows users to tinker with geometric objects. In my regular work day, it is very possible to find me lost in my thoughts playing with Sketchpad, trying to create beautiful shapes with isosceles and right triangles. The ability to drag shapes while changing their dimensions and orientation is really fun.
I don’t know if this rings true for you, but it happens to me a lot: Casual play without a specific goal in mind leads me to discover something new. I still remember the first time I played with a Rubik’s Cube, and I got a whole side of the same color. I honestly had no idea how I had done it! It was only when I tried to undo my moves and repeat the process that I understood better how the cube worked. I think the ability to play is what makes puzzles like Rubik’s Cube so powerful and educational. Play, of course, need not be limited to tangible objects. Sketchpad does a terrific job of allowing students to tinker in the virtual realm.
In the interactive Web Sketchpad model below, students are given a fixed basic shape (like a rectangle or a parallelogram) and a set of draggable “dynamic” triangles that can move, grow, and shrink. The goal is to exactly cover the fixed shape with the given triangles with no overlaps. The triangles are color-coded: orange for right, blue for isosceles, and red for equilateral. This makes it easier for students to keep track of which properties are important for each puzzle. Before you read further, try your hand at the collection of 11 challenges in this Web Sketchpad model.
Students are not required to know beforehand the underlying geometric properties of the shapes. Instead, the objective is for students to approach each challenge like they would any puzzle—it’s a game, not a problem. Just by moving the triangles, students figure out how the triangles fit the given shape. With the triangles in place, the question of why the arrangement of triangles works emerges for the student and helps to illuminate a particular geometric property of the given shapes.
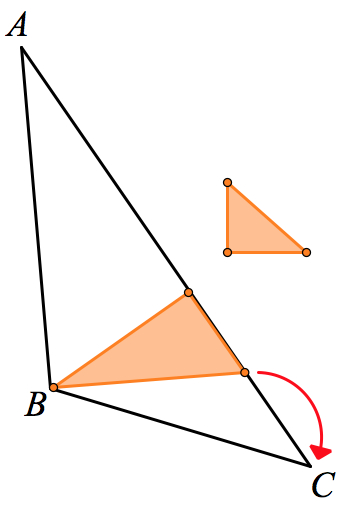
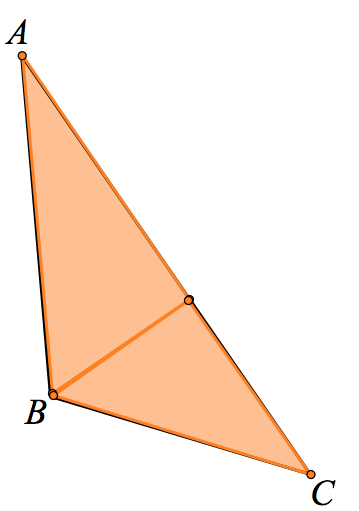
An example from the fourth page of the web sketch will make this idea clear. Students are given a random ΔABC (neither right, isosceles, or equilateral) as the fixed polygon that needs to be decomposed into two right triangles. It requires some playing around to find the answer, but certainly not much. Students start with one right triangle and soon realize that two of its vertices must coincide with two vertices of ΔABC.
From there it is a matter of resizing and rotating the triangles so they fit. The result, shown below, is that the two right angles of the right triangles are adjacent and lying on the base of ΔABC. What information does this convey? Students have just found the height of ΔABC! They also have discovered that two right angles form a line. But can a base have more than one height? Is there another way to fit the triangles together? By playing with the triangles, students will prove or refute their conjectures intuitively through hands-on play.
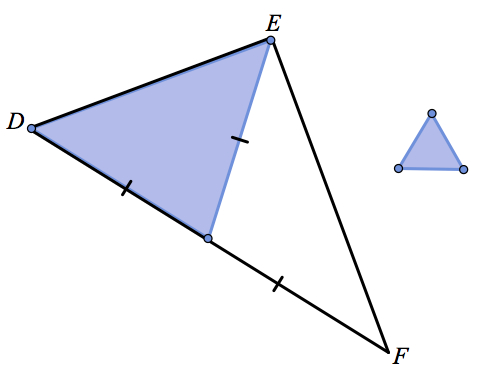
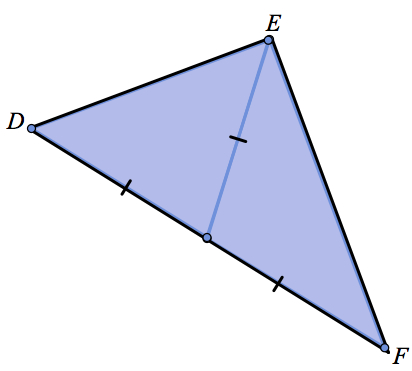
Now, let’s repeat this puzzle with a more complex example. Go to the eighth page of the web sketch. The student is given a right triangle, DEF, to cover and two isosceles triangles to use as pieces. Just like the previous example, the student realizes that two vertices of the isosceles triangle need to coincide with two vertices of the fixed ΔDEF.
With just a little more work, an amazing result emerges: The two isosceles triangles share an equal side in common, and hence the hypotenuse of ΔDEF is divided into two equal parts. Yay! The student has found the median of ΔDEF.
Even if it’s not explicit, the student has uncovered a theorem equivalent to the fifth postulate: a right triangle can be divided into two isosceles triangles with the equivalent sides of the same length. This is a foundational result that we do not expect the student to understand fully, but we do expect her to observe it. And it will feel very natural to the student because she built it. The difficulty of finding the right configuration will give her the idea of how strict this result is: The triangles fit into ΔDEF, but only in a very particular way. But why in such a particular way? The student might say, “Well you see, because you need this side to be equal to this and this equal to that.”
The web sketch has a total of 11 pages, all with underlying geometric results that students will discover through hands-on experimenting. I hope and your students you enjoy the models as much as I did building them!