Estimation is an important mathematical skill, yet we rarely ask students to make estimates that relate to fractions. As part of the Dynamic Number project, we created a “mystery” fraction challenge  that presents a green point somewhere between 0 and 1 on the number line. The point’s location can be represented as a fraction with numerator between 1 and 14 and with denominator between 2 and 15. Students estimate the location of the point, view their estimate on the number line, and then use it to make a new estimate. Through repeated refinement, students pinpoint the mystery fraction’s location.

To try the fraction challenge yourself, use the Web Sketchpad model below (and here). What is its location of the green point, written as a fraction? To make an educated guess, click the Make Fraction tool. You’ll see default values of 1 and 2 appear for the numerator and denominator, respectively. Choosing from the list of numbers from 1-15, click a value for the numerator of your fraction. Then click a value for the denominator. Your fraction should now be plotted and labeled on the number line. If you need to refine your estimate, click the Make Fraction tool again and follow the same procedure to plot a new fraction. The short video at the end of this post demonstrates the use of the tool.

To play again with a new random problem, you can go to pages 2-10 of the websketch and press  New Fraction. On the final 11th page of the websketch, students create their own mystery fraction problems, entering the numerator and denominator values for the mystery fraction while their partner looks away. With the fraction ready, students press Hide Fraction and share the problem.

When I interviewed two fifth graders, Jason and Dylan, to document their strategies for deducing mystery fractions, they sailed right through the problems, pinpointing each fraction with just a few well-chosen guesses. With our interview drawing to a close, I decided to put their fraction skills to the test by changing the list of possible numerators to integers between 13 and 24 and making ¹³/₂₄ the mystery fraction (see below).

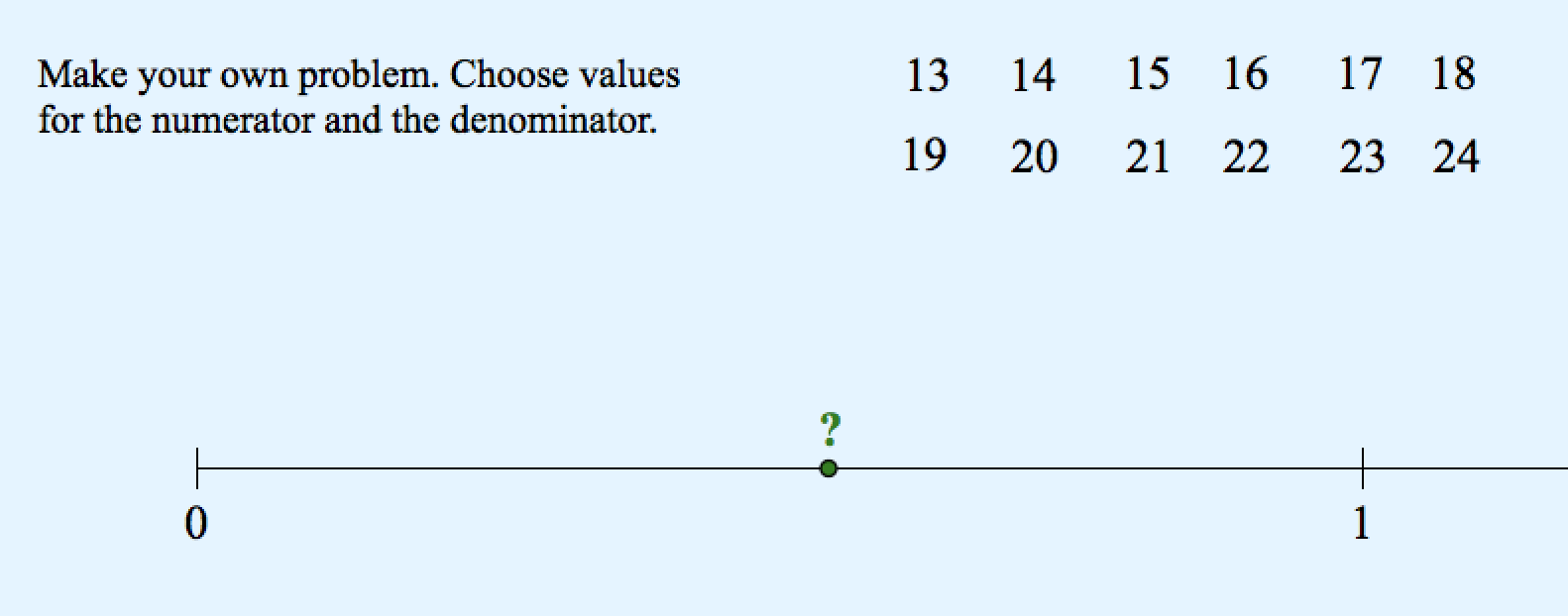
Here is a brief transcript of what happened:

Jason: I think it will be a little bit bigger than one half.

Daniel: Why bigger than a half? How can you tell?

Dylan: Because with the numbers it can’t be one half.

Daniel: Why not?

Dylan: Because 24’s half is 12, and all the odd numbers don’t have half. So 22’s half is 11, 20’s half is 10, 18’s is 9, 16 is 8, and 14 is 7, so there’s none. So half can’t be it.

Daniel: Both of you caught on to my little trick, wanting you to think it was a half, but neither of you got fooled. So what might it be if it’s close to a half?

Jason: Maybe ¹³/₂₄, because ¹²/₂₄ was equivalent to half, so just one more…¹/₂₄ bigger.

I made my mystery fraction nearly one half to trick the students, but Dylan very cleverly surveyed the list of possible numerators and denominators and noticed that no number was twice another. This observation allowed him to swiftly rule out one half as the mystery fraction. Jason then was able to scan the list and pick two numbers that yielded a fraction just a little larger than one half.

I really like the way that Dylan and Jason used not only their knowledge of fractions to solve the problem, but also some elementary number theory to analyze the choices for numerator and denominator!

An annotated list of all our elementary-themed blog posts is here.