In a prior post, I shared some good news: The Connected Geometry high-school curriculum authored by Education Development Center (EDC) is now available for free. I could easily devote every future blog post to a tasty Connected Geometry morsel, but I’ll restrict myself to just a few. The investigation below, taken straight from the curriculum, mixes paper folding with dynamic geometry modeling.

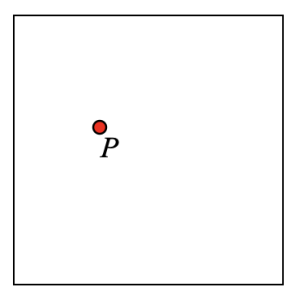
Starting with a square piece of paper, mark an interior point P of your choosing.

One at a time (and unfolding in between) fold each corner to touch point P. Crease carefully each time.

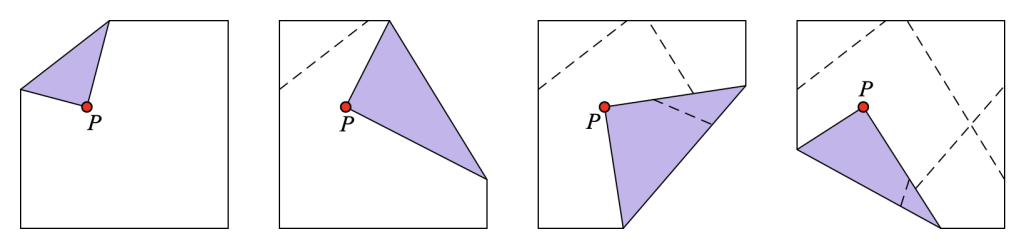
When you are done, the creases will divide the square into several polygonal regions. In the example shown here, the region containing the point P is a hexagon.

Perform the experiment several more times. Does the region containing P always have six sides, no matter where you place P at the start? If you think it does, give a good reason why that should happen. If you think that there will be some other number of sides, give at least one example to support your claim.

Now, consider these questions:

• Find a good reason why the region that contains P must have fewer than nine sides.
• Find a good reason why the region that contains P must have more than three sides.
• Perform some experiments that help you narrow the range of sides even more. What are the minimum and maximum number of sides you find?
• How does the placement of P determine how many sides the polygon will have? Where can you place P to get the minimum and maximum number of sides? (A good way to answer this question is with a picture—perhaps a map of the square, with one color for all the places P can be to produce a hexagon, and a different color for places that produce some other result.)

While paper folding is a great way to begin this investigation, it becomes tedious to start with a fresh square for each new location of point P. Using Web Sketchpad, students can build an interactive version of the situation that allows them to drag point P and watch as the crease lines update automatically. Apart from the ease of being able to move point P, the dynamic model gives students the challenge of modeling geometrically the act of folding the paper and making a crease.

Students can use page 1 of the Web Sketchpad model below (and here) to model the problem. Page 2 shows the completed construction. You can also download the Connected Geometry teacher notes that accompany this activity.