Last week was the fourth session of my spring Advanced Secondary Math Methods class at the University of Pennsylvania. Each year I assign a semester project in which groups of three students use lesson-study techniques—on a small scale—to create, test, refine, teach, evaluate, and document specific shared instructional products, composed of a (possibly multi-day) lesson and its associated assessment. (The term, and the inspiration, comes from an NCTM Research Presession talk by Anne Morris and Jim Hiebert.)

In class we brainstormed about using an interesting and motivating problem to introduce and investigate exponential functions. I imagined the math arising from a problem connecting the keys of a piano to the frequencies of the sounds they produce.

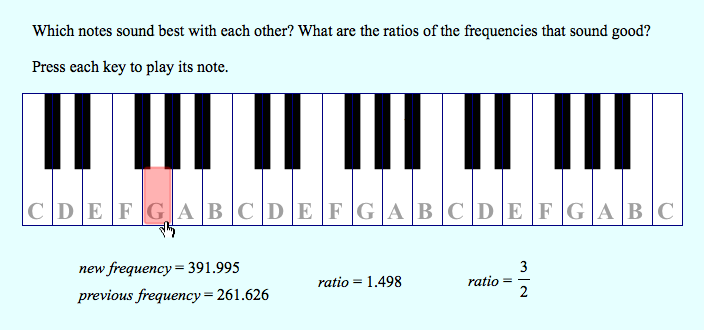
I didn’t want to flesh out a problem in advance, nor did I want us to spend a lot of time floundering to identify an appropriate problem, so I proposed two vague problem topics: a simple one (“Why do certain combinations of notes sound better than other combinations?”) and a significantly more challenging one (“How should you tune a piano to enable as many nice-sounding combinations as possible?”). To situate our brainstorming, I prepared a sequence of ten questions that started with the concept of an octave, developed the idea that harmonious sounds result from frequencies whose ratios can be represented by small integers, and eventually led to the idea of dividing the octave into evenly-spaced steps that include as many harmonious ratios as possible.

The brainstorming was extremely useful; we generated lots of questions, ideas, and implementation suggestions. It was immediately clear that this activity wouldn’t work in a silent classroom; we had to have music, by playing a song to introduce the activity, and by providing a way for students to listen to different frequencies together to find combinations that sound particularly nice. We talked about instruments, and decided the piano presents mathematical advantages (with its one-to-one correspondence between keys and frequencies) but a stringed instrument is a simpler and more easily understood mechanism. While asking students to bring musical instruments to school was a good idea, our methods class nixed it because there might not be any musicians in some classes, and even if there were musicians it didn’t seem smart to ask them to put expensive instruments at risk for the purpose of the lesson.

This discussion inspired me, the next day, to see how hard it would be to create an “instrument” just for this purpose. Here’s what I built, using a piece of scrap wood, some nails and screws, and some picture wire. You tune it with a screwdriver, and you can mark fractions of the length of the strings: 1/2, 1/3, 2/3, 1/4, 3/4, and 4/5. If I were doing this with a class, I’d have students mark the fractional distances with tape or sticky notes so I could remove them before the next class. Note, by the way, that a length of 2/3 produces a frequency of 3/2 of the fundamental — in musical terms, a fifth.

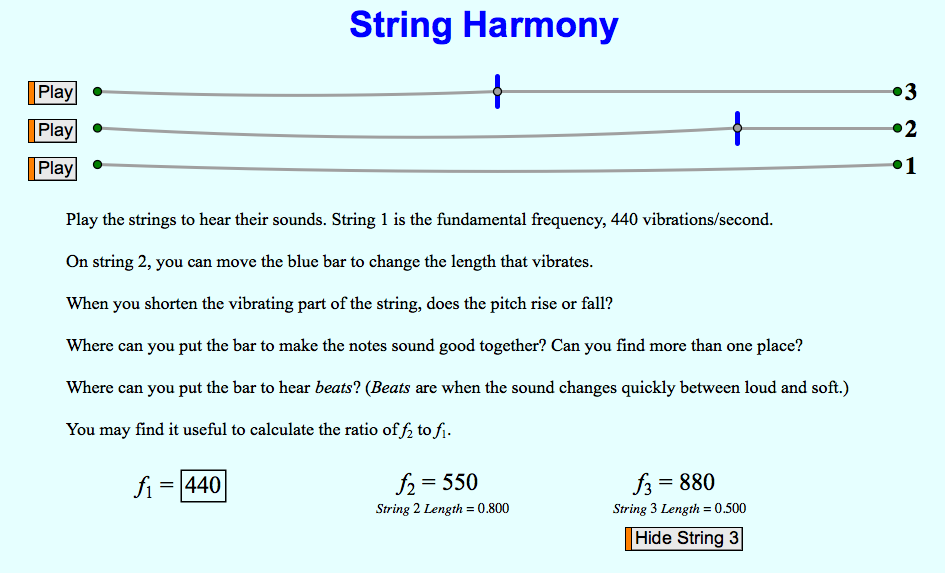
The device is cheap and easy to make, but keeping it in tune is a bit tricky, and hearing the difference between consonant and dissonant plucked notes requires close attention, so it was a natural next step to create a sketch (available for both desktop and iPad) to make the hard parts easy. Students can choose various frequencies to play together, adjust them till they sound “nice,” and then record the frequencies and their ratio in a table. In this way they can discover various harmonies and the ratios that produce them.

Now it’s time to move to the piano, with its nice correspondence between keys and frequencies. It became clear, through our class discussion, that the starting point needed to be a piano that has only A keys, so we can play the keys from A0 through A7, having tuned A4 to 440 hertz. Here again students can make a table to see the pattern of the numbers, and they can graph it in both dynagraph and Cartesian form. This is the perfect time to ask them to describe a function that they could use to find the frequency of A10, or A15, or A4, and to figure out how to describe this function both in words and as a formula. (An important point in this class discussion is to validate both the rule expressed in words and the algebraic formula as appropriate representations of this function—as is the Sketchpad model as well.)

So this is great—-students have defined a function that links piano keys with the frequencies they produce. But we don’t have enough keys to play actual songs, because all our intervals are octaves, based on a ratio of 2:1. This is a new, more advanced problem: how can we divide up the octave intervals in a way that lets us play our favorite harmonies and melodies, the ones defined by small-integer ratios?

Unfortunately, we didn’t have time, in Methods class, even to brainstorm this follow-up problem, though it seems to me that it could be an excellent shared instructional product of its own. And for the first shared instructional product I’ve described here, we shared the brainstorming, but the draft of the worksheet and Sketchpad document are mine alone, without the benefit (yet) of any collaborators. (I haven’t finished the Teacher Notes yet either.) But I think the activity will be fun for students, I encourage you to try it out, and I’d love to have your collaboration, by telling me how it went and how it can be improved.